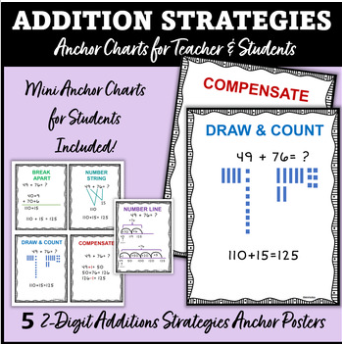
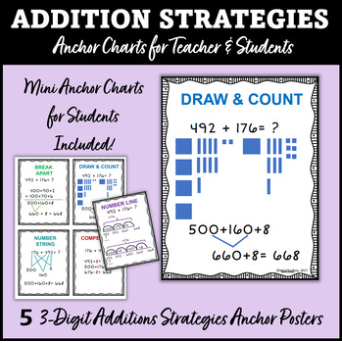
When it comes to teaching addition, no single strategy works perfectly for every student. Providing students with multiple ways to approach addition gives them the freedom to choose the method that feels easiest to them.
Teaching addition through a variety of strategies is key to building math fluency in young learners. Each strategy offers a different approach to problem-solving and by learning different strategies, it will allow for students to have more flexibility thinking and deeper understanding of numbers. Below, I’ll dive into eight essential addition strategies and explain why each is so important for developing foundational math skills in elementary students.
1. Making Ten is one of the most valuable strategies, especially as students move into two-digit addition. The goal is to find combinations that add up to ten, making the addition process smoother. For instance, when adding 8 + 5, students can see that it’s easier to think of 8 + 2 to make 10, then add the remaining 3 to reach 13. This method helps students think strategically, as they can begin looking for number pairs that make ten—a critical concept that supports future skills in regrouping and place value. The strategy of making ten can be effectively visualized with ten frames, where students can see and manipulate groups of ten to reinforce the concept.
2. Doubles Addition is a foundational mental math strategy where students add two identical numbers, such as 4 + 4 or 9 + 9. Learning doubles builds fluency because these additions can be memorized, allowing students to quickly retrieve answers and use doubles as building blocks for larger addition problems. For example, once they know that 6 + 6 is 12, they can also solve 6 + 7 by reasoning that it’s “one more than the double.” This ability to “anchor” around known facts simplifies addition and makes the process less intimidating for young learners.
3. Doubles Plus One (and plus two) is a slight extension of the doubles strategy and is useful for students as they begin working with numbers that differ by just one. In this strategy, if students know that 7 + 7 equals 14, they can find 7 + 8 by adding 7 + 7 and then “plus one” to get 15. Teaching doubles plus one helps students start to break down addition problems into manageable steps and see the connections between numbers. This strategy can easily be demonstrated with counters or a number line, showing that adding one to a known fact makes finding the sum a logical extension.
4. Break Apart (Decomposition) is another essential strategy that helps students see numbers as sums of their parts. For example, when adding 23 + 45, they can decompose each number into tens and ones: 20 + 40 and 3 + 5, which makes the process more manageable. This approach emphasizes place value and allows students to handle larger numbers by focusing on parts they’re more comfortable with. Decomposition is particularly useful because it’s a skill they’ll use frequently in higher math, especially with subtraction, multiplication, and division.
5. Using a Number Line to add provides a visual and interactive way for students to understand addition as “moving forward” on the line. For instance, to solve 6 + 3, students can start at 6 and make three “jumps” forward, landing on 9. This approach reinforces the concept of addition as a form of progression and helps students develop a mental model for counting on. It’s a versatile tool because it can also support subtraction by moving backward, and the number line method can easily transition to more abstract mental math strategies as students become more comfortable.
Need Anchor Charts? Check out these resources!
6. Compensation is a strategy that involves adjusting numbers to simplify addition. This technique can be helpful when adding numbers that are close to a friendly number, like 50 or 100. For example, instead of adding 29 + 45, students can round 29 up to 30, add it to 45, then subtract the extra 1 they added. This approach not only teaches flexibility but also helps students understand that math is fluid—sometimes, adjusting numbers can make a problem easier. Compensation encourages an intuitive understanding of numbers and prepares students for mental math in everyday situations.
7. Using Manipulatives like tens and ones blocks gives students a tactile, hands-on experience of addition. By physically grouping blocks into tens and ones, students can “build” numbers and see how combining them creates new sums. For instance, to add 23 + 14, students can put together two sets of tens and then combine the ones. This strategy is crucial for students who need a visual and physical representation of numbers to solidify their understanding of place value and regrouping. Manipulatives are especially effective for young students as they transition from concrete to abstract thinking.
8. Number Strings are an approach where students add a sequence of smaller numbers in steps, like solving 8 + 6 + 7 by turning it into 10 + 10 + 1. This strategy helps students see addition as a process that can be broken down and rearranged. Number strings build confidence by showing students that there are multiple ways to approach a problem and that they can use smaller, easier-to-manage numbers to reach their answer. This method is particularly helpful in developing flexible thinking and preparing students for more complex addition and multi-step problems.
Conclusion
Teaching students each of these strategies provides students with a toolkit for tackling addition problems in ways that feel easy and logical to them. The goal isn’t just for students to get the right answer but to understand the how and why behind their approach, laying a strong foundation for all their math learning ahead. With these strategies, you’re equipping students not just to add numbers but to think flexibly, approach challenges creatively, and build lasting math skills.